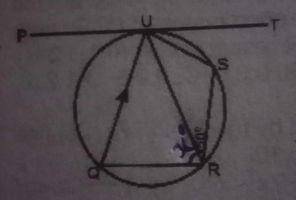
In the figure, PT is tangent to the circle at U and QU/RS if TUR = 35º and SRU = 50º find x
95o
85o
50o
35o
Correct answer is A
Since QRU= Xo
RSU = Xo, But RSU = 180o - (50o + 35o)
= 180o - 85o
= 95o
x = 95o

In the diagram, QP//ST:PQR = 34o qrs = 73o and Rs = RT. Find SRT
68o
102o
107o
141o
Correct answer is B
Construction joins R to P such that SRP = straight line
R = 180o - 107o
< p = 180o - (107o - 34o)
108 - 141o = 39o
Angle < S = 39o (corr. Ang.) But in \(\bigtriangleup\)SRT
< S = < T = 39o
SRT = 180 - (39o + 39o)
= 180o - 78o
= 102o
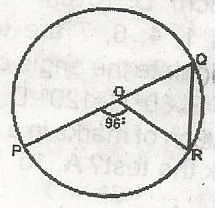
84o
48o
45o
42o
Correct answer is B
OQ = OR = radii
< ROQ = 180 - 86 = 84o
\(\bigtriangleup\)OQR = Isosceles
R = Q
R + Q + 84 = 180(angle in a \(\bigtriangleup\))
2R = 96 since R = Q
R = 48o
ORQ = 48o
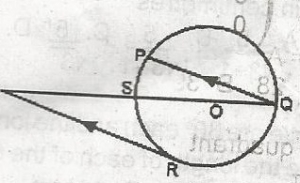
In the diagram, PQRS is a circle with O as centre and PQ/RT. If RTS = 32°. Find PSQ
32o
45o
58o
90o
Correct answer is C
< PSO = \(\frac{1}{2}\) < SOQ = \(\frac{1}{2}\)(180) = 90°
< RTS = < PQS = 32° (Alternative angle)
< PSQ = 90 - < PSQ = 90° - 32°
= 58°
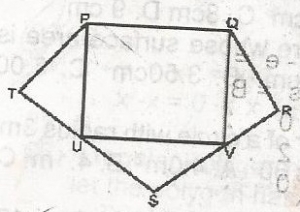
18o
54o
90o
108o
Correct answer is B
The total angle in a regular pentagon = \((2(5) - 4) \times 90\)
= \(6 \times 90 = 540°\)
Each interior angle = \(\frac{540}{5} = 108°\)
While the interior of a quadrilateral = \(\frac{360}{4} = 90°\)
\(PTU + TPU + TUP = 180°\)
\(108° + (180° - 90°) + TUP = 180°\)
\(TUP = 180° - (108° + 18°) = 54°\)
JAMB Subjects
Aptitude Tests