
Further Mathematics Questions and Answers
Test your knowledge of advanced level mathematics with this aptitude test. This test comprises Further Maths questions and answers from past JAMB and WAEC examinations.

Test your knowledge of advanced level mathematics with this aptitude test. This test comprises Further Maths questions and answers from past JAMB and WAEC examinations.
In how many ways can a committee of 3 women and 2 men be chosen from a group of 7 men and 5 women?
500
350
720
210
Correct answer is D
For choosing, its different 'combinations'
Options - 7 men, 5 women
To pick - 2 men, 3 women
∴ The number of ways to choose a committee of 3 women and 2 men from a group of 7 men and 5 women is:
=\(^5C_3 \times ^7C_2\)
=\(\frac{5\times4\times3}{3\times2}\times\frac{7\times6}{2\times1}\)
=\(10\times21\)
=210
2.0
1.4
1.8
1.6
Correct answer is B
n = 5
x̄ = \(\frac{∑x}{n} = \frac{85 + 84 + 83 + 86 + 87}{5} = \frac{425}{5} = 85\)
| \(x\) | \(x - x̄\) | \((x - x̄)^2\) |
| 85 | 0 | 0 |
| 84 | -1 | 1 |
| 83 | -2 | 4 |
| 86 | 1 | 1 |
| 87 | 2 | 4 |
| \(\Sigma(x - x̄)^2 = 10\) |
\(S.D = √\frac{∑(x - x̄ )}{n} = √\frac{10}{5}\)
∴ S.D = √2 =1.4
\(5\frac{2}{5}\)
\(4\frac{1}{5}\)
\(13\frac{1}{2}\)
\(6\frac{3}{4}\)
Correct answer is A
Sum to infinity of a G.P when /r/ < 1 = \(\frac{a}{1 - r}\)
a = \(\frac{9}{2},r = \frac{T_2}{T_1} = \frac{3}{4} + \frac{9}{2}\)
r = \(\frac{3}{4} \times \frac{2}{9} = \frac{1}{6}\)
\(S_∞ = \frac{\frac{9}{2}}{1-\frac{1}{6}} = \frac{\frac{9}{2}}{\frac{5}{6}} = \frac{27}{5}\)
\(\therefore S_∞ = 5\frac{2}{5}\)
2.0
3.0
2.5
4.0
Correct answer is C
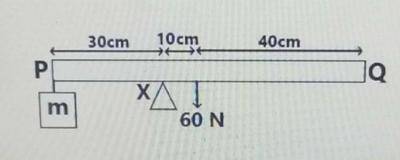
∑ clock wise moments =∑ anti clock wise moments
= 60 x 10 = 30 x m
= 600 = 30 m
m = \(\frac{600}{30} = 20 N\)
W = mg ==> m = \(\frac{W}{g}\)
∴ m = \(\frac{20}{10} = 2kg\)
If \(f : x → 2 tan x\) and \(g : x → √(x^2 + 8), find ( g o f )(45^o)\)
4
2√3
6
3√2
Correct answer is B
\(f : x → 2 tan x\)
\(g : x → √(x^2 + 8)\)
\(( g o f ) = √((2 tan x)^2 + 8)\)
\(( g o f )(45º) = √((2 tan 45º)^2 + 8)\)
= √(4 + 8) = √12
= √(4 x 3)
∴ 2√3