170o
192o
177o
182o
Correct answer is B
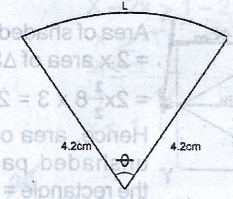
Length of arc, L = 21.4 - 2 x 4.2cm
= 21.4 - 8.4
= 13cm
But L = θ360o x 2πr
i.e 13 = θ360o x 2 x 227 x 4.2
= 13 x 360o x 7
= θ x 2 x 22 x 4.2
θ = 13×360o×744×4.2
= ≈ 177.27o
≈ 177o (to the nearest degree)

2 : 3 : 4
3 : 4 : 5
4 : 5 : 6
5 : 6 : 7
Correct answer is D
m + n = 110o, (n + r) = 130o
(m + n) = 120o
then, r = 130o - n
and;
m + (130^o - n) = 120o
m - n = -10o
2m + (n + r) = 110 + 120 = 230
2m + 130 = 230
2m = 230 - 130
m = 1002 = 50o
n = 110o - 50o
= 60o
r = 130o - 60o = 70o
Hence, the ratio m : n : r
= 50 : 60 : 70
= 5 : 6 : 7
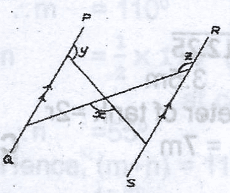
In the diagram, PQ//RS. Find x in terms of y and z
x = 240o - y - z
x = 180o - y - z
x = 360o + y -z
x = 360o - y - z
Correct answer is D
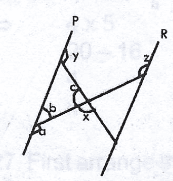
In the diagram,
a = z (alternate angles)
b = 180o - a (angles on a straight line)
b = 180o - z
c = 180o - x (angles on a straight line)
y = b + c (sum of oposite interior angles)
y = 180o - z + 180o - x
y = 360o - z - x
x = 360o - y - z
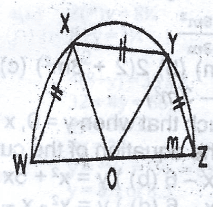
90o
60o
45o
30o
Correct answer is B
In the diagram, < WOZ = 180o (angle on a straight line)
< WOX = < XOY = < YOZ
(|WX| = |XY| = |YZ|)
180o3 = 60o
= 60o
M + m =2m (base angles of isosceles △, |OY| and |OZ| are radii)
< YOZ + 2m (base angles of a △)
60o + 2m = 180o (sum of a △)
60o + 2m = 180o
2m = 180o - 60o
2m = 120o
m = 120o2
= 60o
WAEC Subjects
Aptitude Tests
