A piece of thread of length 21.4cm is used to form a sector ...
A piece of thread of length 21.4cm is used to form a sector of a circle of radius 4.2cm on a piece of cloth. Calculate, correct to the nearest degree, the angle of the sector. [Take π=227]
A.
170o
B.
192o
C.
177o
D.
182o
Correct answer is B
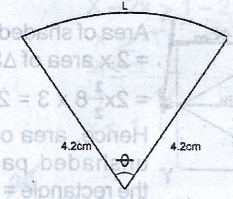
Length of arc, L = 21.4 - 2 x 4.2cm
= 21.4 - 8.4
= 13cm
But L = θ360o x 2πr
i.e 13 = θ360o x 2 x 227 x 4.2
= 13 x 360o x 7
= θ x 2 x 22 x 4.2
θ = 13×360o×744×4.2
= ≈ 177.27o
≈ 177o (to the nearest degree)
Similar Questions
Simplify (6 - x - x2) ÷ (x2 - 4) ...
If N225.00 yields N27.00 in x years simple interest at the rate of 4% per annum, find x ...
The determination of the matrix \(\begin{pmatrix} 1 & 3 & 3 \\ 4 & 5 & 6\\ 2 & 0...
If x=y2,evaluate\(\left(\frac{x^{3}}{y^{3}}+\frac{1}{2}\right) \div \left(\frac{1}{2}...
A cone with the sector angle of 45° is cut out of a circle of radius r of the cone....