
In ΔABC above, BC is produced to D, /AB/ = /AC/ and ∠BAC = 50o. Find ∠ACD
50o
60o
65o
100o
115o
Correct answer is E
ACB = ABC [Base angles of isoceles triangle]
ACB + ABC + 50o = 180o[sum of angles in a triangle]
ACB + ABC = 180o - 50o = 130o
ACD + ACB = 180o[sum of ∠s on a straight line]
ACD + 65o = 180o
ACD = 180o - 65o = 115o
Which of the following angles is an exterior angle of a regular polygon?
95o
85o
78o
75o
72o
Correct answer is E
The formula for the exterior angle of a regular polygon = \(\frac{360}{n}\).
Among the options, only 72° is divisible by 360° to give an integer.
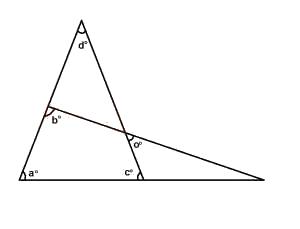
In the diagram above, the value of angles b + c is
180o
90o
45o
ao
do
Correct answer is A
a + c + d - 180o[sum of angles in triangle]
x = a[vertical opp. ∠s]
y + x + d = 180o[sum of ∠s in a Δ]
y + a + d = 180o
y + a + d = a + c + d
y = c
but b + y = 180o [sum of ∠s on a straight line ]
b + c = 180o; Ans = A = 180o
The locus of a point which is equidistant from two given fixed points is the
Perpendicular bisector of the straight line joining them
Angle bisector of the straight lines joining the points to the origin
Perpendiculars to the straight line joining them
Parallel-line to the straight line joining them
A line making an acute angle with the line joining the two points
Correct answer is A
No explanation has been provided for this answer.
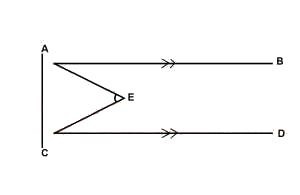
In the diagram above, AB//CD, the bisector of ∠BAC and ∠ACD meet at E. Find the value of ∠AEC
30o
45o
60o
75o
90o
Correct answer is E
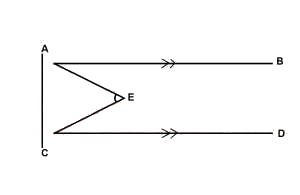
Ae is a bisector of BAC => EAC = 45o
CE is the bisector of ACD => ACE = 45o
AEC = 180o - [EAC + ACE]
= 180o - (45o + 45o) = 180o - 90o = 90o
WAEC Subjects
Aptitude Tests