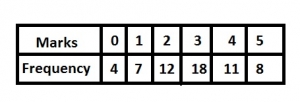
12
23
37
49
Correct answer is D
= 12 + 18 + 11 + 8
= 49 students

1
2
3
4
Correct answer is C
No explanation has been provided for this answer.

3
5
8
18
Correct answer is A
No explanation has been provided for this answer.
Simplify \(\left(\frac{3}{4} - \frac{1}{3}\right)\times 4\frac{1}{3}\div 3\frac{1}{4}\)
\(\frac{5}{9}\)
\(\frac{5}{16}\)
\(\frac{8}{13}\)
\(\frac{16}{5}\)
Correct answer is A
\((\frac{3}{4} - \frac{1}{3}) \times 4\frac{1}{3} \div 3\frac{1}{4}\)
= \((\frac{9 - 4}{12}) \times \frac{13}{3} \div \frac{13}{4}\)
= \(\frac{5}{12} \times \frac{13}{3} \times \frac{4}{13}\)
= \(\frac{5}{9}\)
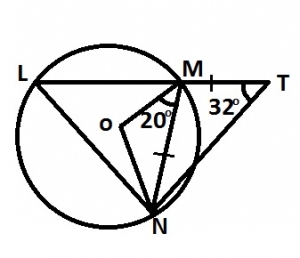
44o
46o
52o
70o
Correct answer is B
< MNT = < MTN = 32°
< NMT = 180° - 2(32°) = 116°
< OMN + < NMT + < LMO = 180°
20° + 116° + < LMO = 180° \(\implies\) < LMO = 180° - 136° = 44°
< LMN = < LMO + < OMN
= 44° + 20° = 64°
< NOM = 180° - 2(20°) = 140°
< NLM = \(\frac{1}{2} \times < NOM = 70°\)
< LNM + < LMN + < NLM = 180°
< LNM + 64° + 70° = 180°
< LNM = 180° - 134° = 46°
WAEC Subjects
Aptitude Tests