If sinx=45, find 1+cot2xcsc2x−1.
132
259
313
411
Correct answer is B
sinx=oppHyp=45
52 = 42 + adj2
adj2 = 25 - 16 = 9
adj = √9 = 3
tanx=43
cotx=143=34
cot2x=(34)2=916
cscx=1sinx
= 145=54
csc2x=(54)2=2516
∴
= \frac{25}{16} \div \frac{9}{16}
= \frac{25}{9}
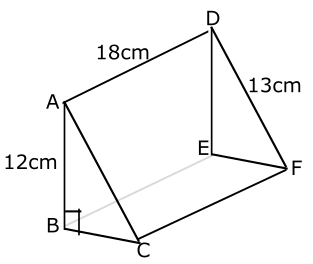
394 cm^3
425 cm^3
268 cm^3
540 cm^3
Correct answer is D
|AC| = |DF| = 13 cm
Using Pythagoras theorem,
|AC|^2 = |AB|^2 + |BC|^2
13^2 = 12^2 + |BC|^2
|BC|^2 = 169 - 144 = 25
|BC| = \sqrt{25}
= 5 cm
Volume of triangular prism = \frac{1}{2} \times base \times length \times height
= \frac{1}{2} \times 5 \times 12 \times 18
= 540 cm^3

In the circle above, with centre O and radius 7 cm. Find the length of the arc AB, when < AOB = 57°
5.32 cm
4.39 cm
7.33 cm
6.97 cm
Correct answer is D
Length of arc = \frac{\theta}{360°} \times 2 \pi r
= \frac{57}{360} \times 2 \times \frac{22}{7} \times 7
= 6.97 cm
| Marks | 1 | 2 | 3 | 4 | 5 |
| Frequency | 2y - 2 | y - 1 | 3y - 4 | 3 - y | 6 - 2y |
The table above is the distribution of data with mean equals to 3. Find the value of y.
5
2
3
6
Correct answer is B
| Marks (x) | 1 | 2 | 3 | 4 | 5 | |
| Frequency (f) | 2y - 2 | y - 1 | 3y - 4 | 3 - y | 6 - 2y | 3y + 2 |
| fx | 2y - 2 | 2y - 2 | 9y - 12 | 12 - 4y | 30 - 10y | 26 - y |
Mean = \frac{\sum fx}{\sum f}
3 = \frac{26 - y}{3y + 2}
3(3y + 2) = 26 - y
9y + 6 = 26 - y
9y + y = 26 - 6
10y = 20 \implies y = 2
JAMB Subjects
Aptitude Tests