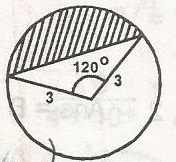
In the figure, the area of the shaded segment is
3π
9√34
3 π−3√34
(√3−π)4
π+9√34
Correct answer is C
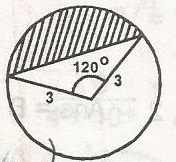
Area of sector = 120360×π×(3)2=3π
Area of triangle = 12×3×3×sin120o
= 92×√32=9√34
Area of shaded portion = 3π−9√34
= 3 π−3√34
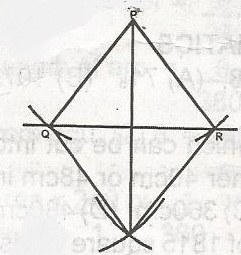
The figure is an example of the construction of a
perpendicular bisector of a given straight line QR
perpendicular from a given point to a given line QR
perpendicular to a line from a given point P on that line
given angle
Correct answer is B
QR is a given line and P is a given point. The construction is the perpendicular from a given point P to a given line QR
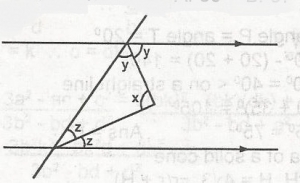
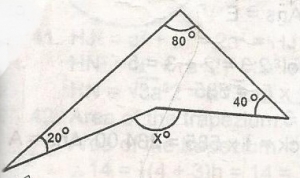
In the figure, find the value of x
110o
100o
90o
80o
Correct answer is C
Z = X = Y = 180o .........(i)
2Z + Y + Y = 190 = 2Z + 2Y = 180
Z + Y = 90o........(ii)
hence x + (z + y) = 180
x + 90o = 180o
x = 180o - 90o
= 90o
JAMB Subjects
Aptitude Tests