In the figure, the area of the shaded segment is
...
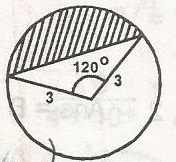
In the figure, the area of the shaded segment is
A.
3π
B.
9√34
C.
3 π−3√34
D.
(√3−π)4
E.
π+9√34
Correct answer is C
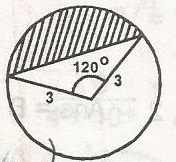
Area of sector = 120360×π×(3)2=3π
Area of triangle = 12×3×3×sin120o
= 92×√32=9√34
Area of shaded portion = 3π−9√34
= 3 π−3√34
Similar Questions
Find the area of the sector OPSQ ...
Subtract 164189 from 186309....
Two ladders of length 5m and 7m lean against a pole and make angles 45° and 60° with the gro...
If the 2nd and 5th terms of a G.P are 6 and 48 respectively, find the sum of the first for term ...
The nth term of the sequence -2, 4, -8, 16.... is given by ...
Two angles of a triangle are 45º each and its longest side is 12cm. Find the length of one...