Simplify: \(\frac{3x - y}{xy} - \frac{2x + 3y}{2xy} + \frac{1}{2}\)
\(\frac{4x + 5y - xy}{2xy}\)
\(\frac{5y - 4x + xy}{2xy}\)
\(\frac{5x + 4y - xy}{2xy}\)
\(\frac{4x - 5y + xy}{2xy}\)
Correct answer is D
\(\frac{3x - y}{xy} - \frac{2x + 3y}{2xy} + \frac{1}{2}\)
= \(\frac{2(3x - y) - 1(2x + 3y) + xy}{2xy}\)
= \(\frac{6x - 2y - 2x - 3y + xy}{2xy}\)
= \(\frac{4x - 5y + xy}{2xy}\)
5.0
4.0
3.0
2.5
Correct answer is A

Diagonal |AC| = (2x + 1)cm
In the diagram,area of \(\Delta\)ABC
is \(\frac{110}{2}\) = \(\frac{1}{2}\) x |AC| x |HB|
55 = \(\frac{1}{2}\) x (2x + 1) x 10
55 = (2x + 1)5
55 = 10x + 5
55 - 5 = 10x
50 = 10x
x = \(\frac{50}{10}\)
= 5.0
Which of the following is used to determine the mode of a grouped data?
Bar chart
Frequency polygon
Ogive
Histogram
Correct answer is D
No explanation has been provided for this answer.
Make K the subject of the relation T = \(\sqrt{\frac{TK - H}{K - H}}\)
K = \(\frac{H(T^2 - 1)}{T^2 - T}\)
K = \(\frac{HT}{(T - 1)^2}\)
K = \(\frac{H(T^2 + 1)}{T}\)
K = \(\frac{H(T - 1)}{T}\)
Correct answer is A
T = \(\sqrt{\frac{TK - H}{K - H}}\)
Taking the square of both sides, give
T2 = \(\frac{TK - H}{K - H}\)
T2(K - H) = TK - H
T2K - T2H = TK - H
T2K - TK = T2H - H
K(T2 - T) = H(T2 - 1)
K = \(\frac{H(T^2 - 1)}{T^2 - T}\)
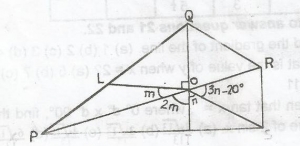
35o
40o
55o
60o
Correct answer is A
In the diagram, QOR + 2m(vertically opposite angles)
So, m + 90° + 2m = 180°
(angles on str. line)
3m = 180° - 90°
3m = 90°
m = \(\frac{90^o}{3}\)
= 30°
substituting 30° for m in
2m + 4n = 200° gives
2 x 30° + 4n = 200°
60° + 4n = 200°
4n = 200° - 60°
= 140°
n = \(\frac{140°}{4}\)
= 35°
WAEC Subjects
Aptitude Tests