Simplify: (\(\frac{3}{4} - \frac{2}{3}\)) x 1\(\frac{1}{5}\)
\(\frac{1}{60}\)
\(\frac{5}{72}\)
\(\frac{1}{10}\)
1\(\frac{7}{10}\)
Correct answer is C
(\(\frac{3}{4} - \frac{2}{3}\)) x 1\(\frac{1}{5}\)
= (\(\frac{9 - 8}{12} \times \frac{6}{5}\))
= \(\frac{1}{12} \times \frac{6}{5}\)
= \(\frac{1}{10}\)
If 23x + 101x = 130x, find the value of x
7
6
5
4
Correct answer is D
23x + 101x = 130x
2 x X1 + 3 x Xo + 1 x X2 + 0 x X1 + 1 x Xo
= 1 x Xo = 1 x X2 + 3 x X1 + 0 x Xo
= X2 + 3x + 0
2x + 3 = x2 + 0 + 1 + x2 + 3x
2x - 3x + x2 - x2 = -3 - 1
- x = -4
x = 4
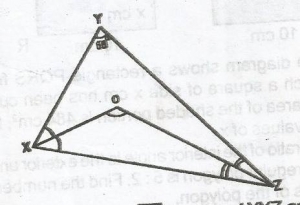
68o
72o
112o
124o
Correct answer is D
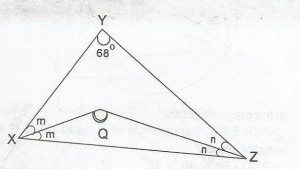
In \(\Delta\) XYZ, 2m + 2n + 68o = 180o
2(m + n) + 68o = 180o...(1)
in \(\Delta\) XOZ, m + n + q = 180o ...(2)
(m + n) = 180o - q...(3)
substituting 180o - q for (m + n) in (1) gives
2(180o - q) + 68o = 180o
360o - 2q = 180o - 68o
360o - 2q = 112o
360o - 112o = 2q
248o = 2q
q = \(\frac{248^o}{2}\)
= 124o
hence, < XOZ = 124o
22cm
17cm
16cm
15cm
Correct answer is B
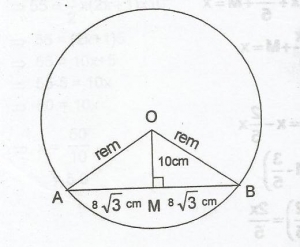
In the diagram
|AM| = |MB| - \(\frac{|AB|}{2}\)
= \(\frac{16\sqrt{3}}{2}\)cm
= 8\(\sqrt{3}\)cm
in \(\Delta\) AMO, r2 = |AM|Z + |MO|2
r2 = (8\(\sqrt{3}\))2
+ 102
= 64 x 3 + 100
= 192 + 100
= 292
r = \(\sqrt{292}\)
17.088cm
17cm
\(\frac{9}{13}\)
\(\frac{11}{26}\)
\(\frac{4}{13}\)
\(\frac{1}{26}\)
Correct answer is C
Two word mathematics is first rewritten as mathematics, since every letter of mathematics appears once in the english alphabet.
Hence the probability that letter selected is from the word mathematics is prob. (matheics) = \(\frac{8}{26}\)
= \(\frac{4}{13}\)
WAEC Subjects
Aptitude Tests