Processing math: 100%

WAEC Mathematics Past Questions & Answers - Page 47

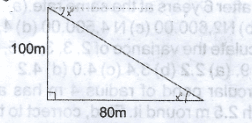
231.

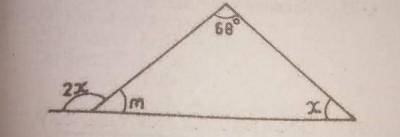
Find the value of m in the diagram

A.

72 $^o$

B.

68 $^o$

C.

44 $^o$

D.

34 $^o$

View answer & explanation

Correct answer is C

2x + m = 180

x + m = 112

x = 122 - m

2(112 - m) + m = 180

224 - 2m + m = 180

224 - m = 180

224 - 180 = m

m = 44 $^o$

232.

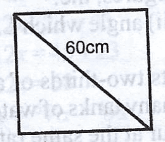
The diagonal of a square is 60 cm. Calculate its peremeter

A.

20 $\sqrt{2}$

B.

40 $\sqrt{2}$

C.

90 $\sqrt{2}$

D.

120 $\sqrt{2}$

View answer & explanation

Correct answer is D

$60^2 + x^2 + x^2$

$360^2 = 2x^2$

$x^2$ = 1800

x = $\sqrt{1800}$

x = 42.4264

x = 42.4264

perimeter = 4x

= 4 x 42.4264

= 169.7056

= 120 $\sqrt{2}$

= 120 $\sqrt{2}$

233.

A stationary boat is observed from a height of 100m. If the horizontal distance between the observer and the boat is 80m, calculate, correct to two decimal places, the angles of depression of the boat from point of observation

A.

36.87 $^o$

B.

39.70 $^o$

C.

51.34 $^o$

D.

53.13 $^o$

View answer & explanation

Correct answer is C

Tan $x^o = \frac{100m}{80}$

Tan $x^o = Tan^{-1} 1.25$

x = 51.34 $^o$

234.

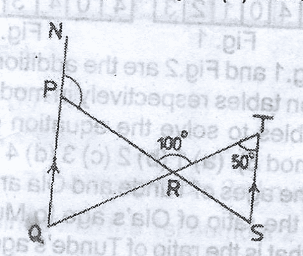
In the diagram, NQ//TS, <RTS = 50 $^o$ and <PRT = 100 $^o$ . Find the value of <NPR

A.

110 $^o$

B.

130 $^o$

C.

140 $^o$

D.

150 $^o$

View answer & explanation

Correct answer is B

< TSR = 180 - (80 + 50)

= 180 - (130)

= 50 $^o$

< QPR = < TSR corresponding < s

< NPR + QPR = < NPR

180 $^o$ - < QPR = < NPR

180 $^o$ - 50 = < NPR

< NPR = 130 $^o$

235.

In a class of 45 students, 28 offer chemistry and 25 offer Biology. If each student offers at least one of the two subjects, calculate the probability that a student selected at random from the class the class offers chemistry only.

A.

$\frac{2}{9}$

B.

$\frac{4}{9}$

C.

$\frac{5}{9}$

D.

$\frac{7}{9}$

View answer & explanation

Correct answer is B

28 - x + x + 25 - x = 45

53 - x = 45

x = 53 - 45

x = 8

chemistry only = 28 - 8

= 20

Probability = $\frac{20}{45}$

= $\frac{4}{9}$

WAEC Subjects

English Language

Agricultural Science

Physical Education

Further Mathematics

Literature in English

Islamic Religious Knowledge

Christian Religious Knowledge

Office Practice

Civic Education

Aptitude Tests

Nigeria General Knowledge Questions and Answers

Personality Test

Microsoft Office Questions and Answers

Quantitative Reasoning Questions and Answers

Spatial Reasoning Questions and Answers

Verbal Reasoning Questions and Answers

Abstract Reasoning Questions and Answers

Puzzles Questions and Answers

Typing Speed and Accuracy Test

Situational Judgement Questions and Answers

Data Interpretation Questions and Answers

Numerical Reasoning Questions and Answers

Logical Reasoning Questions and Answers

View latest jobs in Nigeria today