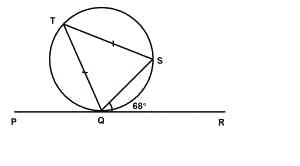
In the diagram, PQR is a tangent to the circle QST at Q. If |QT| = |ST| and ∠SQR = 68°, find ∠PQT.
34o
48o
56o
68o
73o
Correct answer is C
< STQ = < SQR = 68° (alternate segment)
∴ < STQ = 68°
< TQS = \frac{180° - 68°}{2}
= \frac{112}{2} = 56°
\therefore < PQT = 180° - (68° + 56°)
= 180° - 124°
= 56°
22cm
33.5cm
43cm
66cm
115.5cm
Correct answer is C
Perimeter of a sector = 2r + \frac{\theta}{360} \times 2\pi r
= 2(10.5) + \frac{120}{360} \times 2 \times \frac{22}{7} \times 10.5
= 21 + 22
= 43 cm
5cm
6cm
10cm
12cm
15cm
Correct answer is C
Area of rhombus = \frac{pq}{2}
where p and q are the two diagonals of the rhombus.
\therefore 60 = \frac{12 \times q}{2}
6q = 60 \implies q = 10 cm
49.5cm3
99cm3
148.5cm3
297cm3
445.5cm3
Correct answer is C
Volume of a cone = \frac{1}{3} \pi r^2 h
r = 4\frac{1}{2} cm; h = 14 cm
Volume of cone = \frac{1}{3} \times \frac{22}{7} \times \frac{9}{2} \times \frac{9}{2} \times 14
= 297 cm^3
When half- filled, the volume of the water = \frac{297}{2} = 148.5 cm^3
Calculate the total surface area of a cone of height 12cm and base radius 5cm. [Take π = 22/7]
180 5/7cm2
240 2/7cm2
235 5/7cm2
282 6/7cm2
361 3/7cm2
Correct answer is D
No explanation has been provided for this answer.
WAEC Subjects
Aptitude Tests