
130°
115°
58°
68°
Correct answer is C
Alternate segments are equal : R° = 64°
An isosceles triangle has two angles ( Q° & N° ) equal as A°
R° + A° + A° = 180°
2A°= 180 - 64
2A° = 116°
A = 1162
A = 58.
: Q° = 58, N° = 58 and T° = 58
As Alternate segments are equal: T = Q
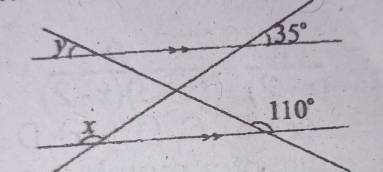
215°
70°
135°
145°
Correct answer is A
sum of angle in a straight line = 180°
35 + m = 180
m = 180 - 35 = 145°
Corresponding angles are congruent i.e equal to each other
Angle X and M are congruent = 145
sum of angle in a straight line = 180°
110 + n = 180
m = 180 - 110 = 70°
Angle Y and N are congruent = 70°
∴∴ ( x + y) = ( 145 + 70)°
(x + y) = 215°
Find, correct to two decimal, the mean of 112, 223, 334, 445, and 556.
3.71
3.70
3.69
3.72
Correct answer is A
The mean = sum of 112, 223, 334, 445, and 556 divided by their quantity.
Mean = ( 112 + 223 + 334 + 445 + and 556 ) / 5
= 3.708 or 3.71( 2 d.p )
π =227
84cm2
80cm2
30cm2
94cm2
Correct answer is B
Area of circle = πr2 = 227 * 72 = 154cm2
Area o Trapezium = (a+b)h2 = (10+21)82 = 124cm2
Area of the region not covered = Area of (Circle - Trapezium)
= 154 - 124
= 30cm2

In the diagram, O is the centre of the circle PQRS, ∠PQR = 72° and OR is parallel to PS. Find ∠ OPS.
18°
108°
54°
36°
Correct answer is D
Angle at the centre O is twice the angle at the circumference.
i.e O = 72° * 2 = 144°
∠OPS = 180 - 144 = 36°
WAEC Subjects
Aptitude Tests