
In the diagram, |QR| = 10cm, PR⊥QS, angle PSR = 30° and angle PQR = 45°. Calculate in meters |QS|
10(1+√3)
20√3
10√3
(10+√3)
Correct answer is A
In Δ PQR,
tan45=PR10⟹PR=10tan45
= 10m
In Δ PRS,
tan30=10RS⟹RS=10tan30
= 10{1√3
= 10√3
PS = 10+10√3
= 10(1+√3)cm
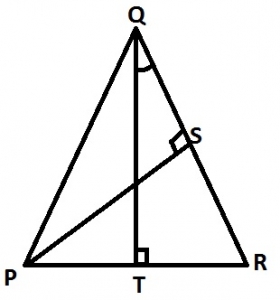
In the diagram, ¯PSand¯QT are two altitudes of ∆PQR. Which of the following is equal to ∠RQT?
∠PQT
∠SRP
∠PQR
∠SPR
Correct answer is D
No explanation has been provided for this answer.
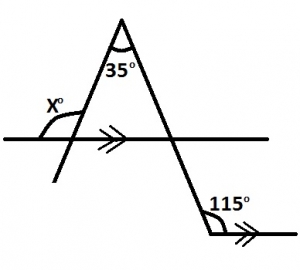
In the diagram, calculate the value of x
35o
80o
100o
115o
Correct answer is C
x - 35° = 65° (corresponding angles)
x = 65° + 35° = 100°
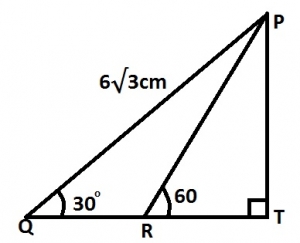
0.3cm
√32cm
3cm
3√3cm
Correct answer is C
In Δ QPT,
\frac{PT}{6\sqrt{3}} = \sin 30°
PT = 6\sqrt{3} \times \frac{1}{2} = 3\sqrt{3} cm
In \Delta RPT,
\frac{PT}{RT} = \tan 60°
\frac{3\sqrt{3}}{RT} = \tan 60°
RT = \frac{3\sqrt{3}}{\sqrt{3}} = 3 cm
If q oranges are sold for t Naira, how many oranges can be bought for p naira?
\frac{p}{2}t
\frac{qt}{p}
\frac{q}{pt}
\frac{pq}{t}
Correct answer is D
q oranges = t naira
1 naira = \frac{q}{t}
p naira = p(\frac{q}{t})
= \frac{pq}{t} oranges
WAEC Subjects
Aptitude Tests