972.
A.
10two
B.
100two
C.
1100two
D.
11000two
Correct answer is D
(1112)2−(1012)2
Difference of two squares
(111−101)(111+101)
= (10)(1100)
= 110002
973.
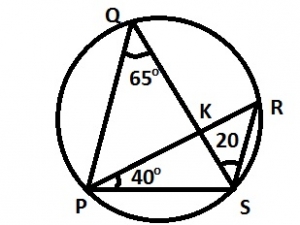

In the diagram, PˆQS=65o,RˆPS=40oandQˆSR=20o,findPˆSQ
A.
85o
B.
60o
C.
55o
D.
45o
Correct answer is C
< QPS = < PRS = 65° (angles in the same segment)
< PSR + 40° + 65° = 180°
< PSR + 105° = 180°
< PSR = 75°
< PSR = < PSQ + < QSR
75° = < PSQ + 20° ⟹ < PSQ = 75° - 20° = 55°
974.
Find the values of x for which 12x2−13x+15 is not defined,
A.
5 or 32
B.
1 or 1513
C.
2 or 15
D.
13 or 15
Correct answer is A
The fraction is undefined when the denominator is equal to zero
2x2−13x+15=02x2−3x−10x+15x(2x−3)−5(2x−3)=0(2x−3)(x−5)=0x=32orx=5
WAEC Subjects
Aptitude Tests