WAEC Mathematics Past Questions & Answers - Page 191

Sin (5x – 28)^o = cos (3x - 50)^o
Since by the trigonometry relation
Sin(5x – 28)^o = cos[90 – (5x – 28)]^o
Hence cos(3x – 50)^o = cos[90 – (5x – 28)]^o
3x – 50 = 90 - (5x-28)
3x – 50 = 90 – 5x + 28
3x + 5x = 90 + 28 + 50
8x = 168
$x = \frac{168}{8}=21^{\circ}$

$23_{five} = X_{ten}; X_{ten} = 2\times 5^1 + 3\times 5^0 = 10 + 3 = 13\\ 101_{three}=P_{ten}; P_{ten} = 1\times 3^2 + 0\times 3^1 + 1\times 3^0=9+0+1=10_{ten}\\ Y = 13+10=23_{ten}$;
Converting to base two
$\begin{matrix} 2 & 23\\ 2 & 11 &R1\\ 2 & 5 & R1\\ 2 & 2 & R1\\ 2 & 1 & R0\\ & 0& R1 \uparrow\\ \end{matrix} \\ =y=10111_2$

$202^2_{three}$when converted to base ten $=(202_3)^2\\ 202_3 = 2 \times 3^2 + 0 \times 3^1 + 2\times 3^0 = 18 + 0 + 2\\ =20_{ten}; (202_3)^2 = (20)^2_{ten} = 400\\ 112^2_{three}$when converted to base ten $= (112_3)^2\\ 112_3 = 1 \times 3^2 + 1 \times 3^1 + 2\times 3^0 = 9+3+2=14_{ten}\\ (112_3)^2 = (14)^2_{ten} = 196_{ten}\\ Evaluate \Longrightarrow 400-196 = 204$
Reconvert to base three
$\begin{matrix} 3 & 204\\ 3 & 69 &R0\\ 3 & 22 & R2\\ 3 & 7 & R1\\ 2 & 2 & R1\\ & 0& R2 \uparrow\\ \end{matrix} \\ =21120_3$

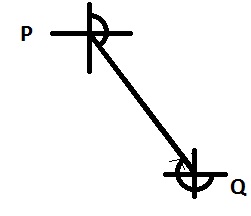
Bearing of Q and P
$180^{\circ} – (360^{\circ}-x)\\ 180^{\circ}-360^{\circ}+x\\ -180^{\circ}+x\\ x-180^{\circ}$

15% loss = N595

$\implies$ (100 - 15)% of CP = N595

85% of CP = N595

CP = $\frac{595 \times 100}{85}$

= N700.00