Given that \sqrt{128}+\sqrt{18}-\sqrt{K} = 7\sqrt{2}, find K,
8
16
32
48
Correct answer is C
\sqrt{128} +\sqrt{18}-\sqrt{k}=7\sqrt{2}\\ \sqrt{2\times 64}+\sqrt{9\times 2} - \sqrt{k} = 7\sqrt{2}\\ 8\sqrt{2} + 3\sqrt{2} - \sqrt{k} = 7\sqrt{2}; 11\sqrt{2} - \sqrt{k} = 7\sqrt{2}\\ -\sqrt{k}=7\sqrt{2}-11\sqrt{2}; -\sqrt{k} = -4\sqrt{2}; \sqrt{k}=4\sqrt{2}\\ =\sqrt{4^2\times 2} = \sqrt{16\times 2}; \sqrt{k}=\sqrt{32}; k = 32
Find the average of the first four prime numbers greater than10
20
19
17
15
Correct answer is D
First four prime numbers greater than 10 are 11, 13, 17, 19
The average = \frac{11+13+17+19}{4}=15
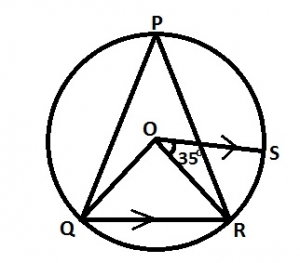
In the diagram, O is the centre of the circle where OS//QR and ∠SOR = 35o
35o
45o
55o
70o
Correct answer is C
∠SOR = ∠ORQ alternate angle.
Also ∠OQR = ∠OQR (Base angle of isosceles)
∠QOR = 108 – 2(35o) = 180 - 70o - 110o ∠QPR = 1/2QOR = 1/2(110o) ∠QPR + 55o (theorem angle at center is twice angle at circumference)
WAEC Subjects
Aptitude Tests