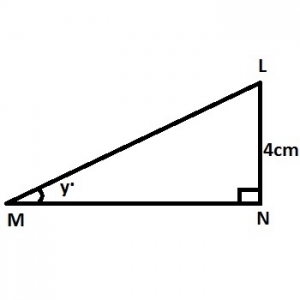
IN the diagram, |LN| = 4cm, LNM = 90o and tan y = 2/3. What is the area of the ∆LMN?
24cm2
12cm2
10cm2
6cm2
Correct answer is B
\(tan \hspace{1mm}y = \frac{opp}{adj}=\frac{2}{3}\Rightarrow \frac{2}{3}=\frac{
LN}{MN}=\frac{4}{MN}\\
∴ MN = \frac{12}{2}=6\\
Area \hspace{1mm}of \hspace{1mm}\Delta LMN = \frac{1}{2}\times MN\times LN=\frac{1}{2}\times 6\times 4=12cm\)
\(5\frac{1}{2}cm\)
\(4\frac{2}{3}cm\)
\(3\frac{1}{2}cm\)
\(2\frac{1}{3}cm\)
Correct answer is D
\(r=\frac{\theta \times l}{360^{\circ }}\\
r=?, \theta =60^{\circ }\), l = radius of the orginal sector of a circle = 14cm
\(r=\frac{60\times 14}{360}=\frac{7}{3}=2\frac{1}{3}cm\)
2.5cm
3.0cm
3.5cm
4.0cm
Correct answer is D
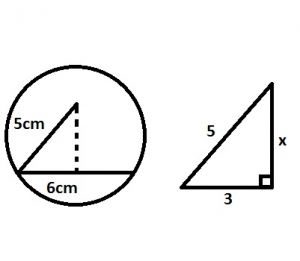
\(5^{2}=3^{2}+x^{2}\\
25-9=x^{2}\\
16=x^{2}\Rightarrow x=4.0\)
The area of a square field is 110.25m2. Find the cost of fencing it round at N75.00 per meter square
N1,575.00
N3,150.00
N4,734.30
N8,268.75
Correct answer is D
N75.00 x 110.25 = N8,268.75
If \(8^{x+1}=\frac{1}{4}\), find x
\(-\frac{5}{3}\)
-1
\(-\frac{1}{3}\)
\(\frac{1}{3}\)
Correct answer is A
\(8^{x+1}=\frac{1}{4}\\
2^{3(x+1)}=2^{-2}\Rightarrow 3(x+1)=-2\Rightarrow 3x+3=-2\\
3x=-5\Rightarrow x=\frac{5}{3}\)
WAEC Subjects
Aptitude Tests