If x% of 240 equals 12, find x
x = 1
x = 3
x = 5
x = 7
Correct answer is C
x% of 240 = 12
\(\frac{x}{100} \times 240 = 12\)
x = \(\frac{12 \times 100}{240}\)
x = 5
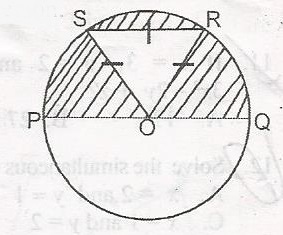
43.36cm2
32.072
18.212
6.932
Correct answer is C
Area of shaded portion = Area of semicircle
Area of \(\bigtriangleup\) RSO
Area of semicircle = \(\frac {\pi r^2}{2} = \frac{22\times 4 \times 4}{7 \times 2}\)
= 25.14cm2; Area of \(\bigtriangleup\)RSO
=\(\sqrt{s(s - 1)(s - b)(s - c)}\); where
s = \(\frac{a + b + c}{2}\)
s = \(\frac{4 + 4 + 4}{2}\)
= 6cm
= \(\sqrt{6(6 - 4)(6 - 4) (6 - 4)}\)
= \(\sqrt{6(2) (2) (2)}\)
= \(\sqrt{18}\) = 6.93cm2
Area of shaded region
= 25.14 - 6.93
= 18.21cm2
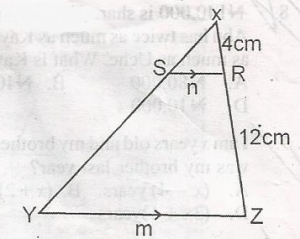
In the diagram, |XR| = 4cm
|RZ| = 12cm, |SR| = n, |XZ| = m and SR||YZ. Find m in terms of n
m = 2n
m = 3n
m = 4n
m = 5n
Correct answer is C
\(\frac{n}{4} = \frac{m}{4 + 12}\)
\(\frac{n}{4} = \frac{m}{16}\)
4m = 16n
\(\frac{4m}{4} = \frac{16n}{4}\)
m = 4n
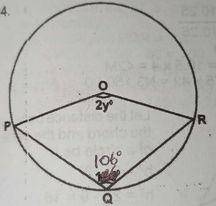
In the diagram, O is the centre of the circle and < PQR = 106º, find the value of y
16
37
74
127
Correct answer is C
2y + Reflex angle = 360
Reflex angle = 360 - 2y
360 - 2y = 2(106)(angles at a centre = 2ce < of circum.)
360 - 212 = 2y
148 = 2y
y \(\frac{148}{2}\)
y = 74o
If the ratio x:y = 3:5 and y:z = 4:7, find the ratio x:y:z
15 : 28 : 84
12 : 20 : 35
3 : 5 : 4
5 : 4 : 7
Correct answer is B
x : y = 3.5, y : z = 4 : 7
x = 3 x 4 = 12
y = 5 x 4 = 20
y = 4 x 5 = 20
z = 7 x 5 = 35
then x : y : z = 12 : 20 : 35
WAEC Subjects
Aptitude Tests