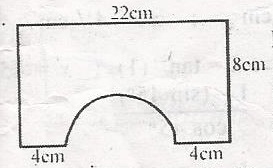
44cm2
99cm2
154cm2
165cm2
Correct answer is B
Area of remaining = Area of rectangle = Area of semi-circle
22 x 8 - 12xar2
ehere r - 142cm = 7cm
Area of remaining = 176 - 12 x 224 x 7 x 7
= 176 - 77
= 99cm2
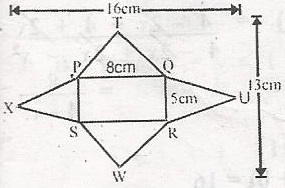
The diagram is a net right rectangular pyramid. Calculate the total surface area
208cm2
112cm2
92cm2
76cm2
Correct answer is C
Total surface area = sum of the area of the △ S + Area of the rectangle
= 2 x Area of △ PTQ + 2 x Area of △ QUR + Area of rectangle PQRS
2 x 12(8 x 4) + 2 x 12(5 x 4) + 8 x 5
= 32 + 20 + 40
= 92cm2
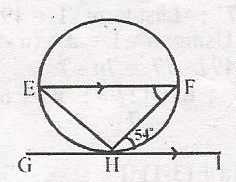
In the diagram, GI is a tangent to the circle at H. If EF//GI, calculate the size of < EHF
126o
72o
64cmo
32cmo
Correct answer is B
F = 54o (Alternate triangle angles)
< GHE = F = 54o(Angle between a chord and a tangent = angles in the alternate segment)
Now, < GHE + < EHF + < IHF = 180o(Angles on a straight line)
54o + < EHF + 54o = 180o
< EHF = 180o - 108o
= 72o

The shaded portion in the diagram is the solution of
x + y ≤ 3
x + y < 3
x + y > 3
x + y ≥ 3
Correct answer is B
Using xa+yb < 1for the equation of the time
where a = intercept on x-axis and b = intercept on y - axis
x3+y3=1
= x+y3=1
= x + y < 3
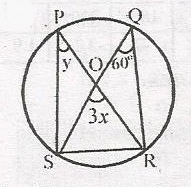
110o
100o
80o
70o
Correct answer is B
3x = 2 x 60 = 2y (Angle at centre = 2 x angle at circumference)
3x = 2 x 60
x = 2×603 = 40o
2 x 60 = 2y
y = 60o
x + y = 40 + 60
= 100o
WAEC Subjects
Aptitude Tests