WAEC Mathematics Past Questions & Answers

566.

In the diagram /Pq//TS//TU, reflex angle QPS = 245^o angle PST = 115^o, , STU = 65^o and < RPS = x. Find the value of x

80^o

70^o

60^o

50^o

View answer & explanation

Correct answer is D

< QPR = < STU = 65^o (Corresponding angles)

245 + < QPR = x = 360^o (< s at a point)

i.e. 245 + 65 + x = 360

x = 360 - (245 + 65)

x = 360 - 310

x = 50^o

567.

The venn diagram shows the number of students in a class who like reading(R), dancing(D) and swimming(S). How many students like dancing and swimming?

View answer & explanation

Correct answer is A

student that like swimming = x + 2

where 2 is the number of students who like reading, dancing and swimming. To find x from the venn diagram of swimming

6 +3 + 2 + x = 16

11 + x = 16

x = 16 - 11 = 5

no. of students that like dancing and swimming

x + 2 = 5 + 2 = 7

568.

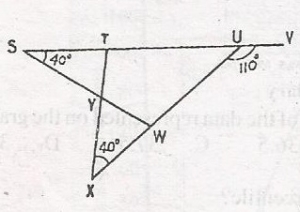
In the diagram, STUV is a straight line. < TSY = < UXY = 40^o and < VUW = 110^o. Calculate < TYW

150^o

140^o

130^o

120^o

View answer & explanation

Correct answer is A

< TUW = 110^o = 180^o (< s on a straight line)

< TUW = 180^o - 110^o = 70^o

In \(\bigtriangleup\) XTU, < XUT + < TXU = 180^o

i.e. < YTS + 70^o = 180

< XTU = 180 - 110^o = 70^o

Also < YTS + < XTU = 180 (< s on a straight line)

i.e. < YTS + < XTU - 180(< s on straight line)

i.e. < YTS + 70^o = 180

< YTS = 180 - 70 = 110^o

in \(\bigtriangleup\) SYT + < YST + < YTS = 180^o(Sum of interior < s)

SYT + 40 + 110 = 180

< SYT = 180 - 150 = 30

< SYT = < XYW (vertically opposite < s)

Also < SYX = < TYW (vertically opposite < s)

but < SYT + < XYW + < SYX + < TYW = 360

i.e. 30 + 30 + < SYX + TYW = 360

but < SYX = < TYW

60 + 2(< TYW) = 360

2(< TYW) = 360^o - 60

2(< TYW) = 300^o

TYW = \(\frac{300}{2}\) = 150^o
< SYT