What is the perpendicular distance of a point (2, 3) from the line 2x - 4y + 3 = 0?
\(\frac{\sqrt{5}}{2}\)
\(\frac{\sqrt{5}}{20}\)
\(\frac{5}{\sqrt{13}}\)
6
Correct answer is A
2x - 4y + 3 = 0
Required distance = \(\frac{(2 \times 2) + 3(-4) + 3}{\sqrt{2^2} + (-4)^2}\)
= \(\frac{4 - 12 + 3}{\sqrt{20}}\)
= \(\frac{-5}{-2\sqrt{5}}\)
= \(\frac{\sqrt{5}}{2}\)
The locus of a point which is equidistant from two given fixed points is the
perpendicular bisector of the straight line joining them
parallel line to the straight line joining them
transverse to the straight line joining them
angle bisector of 90o which the straight line joining them makes with the horizontal
Correct answer is A
No explanation has been provided for this answer.
240o
210o
150o
60o
Correct answer is B
Using sinØ = \(\frac{6√3}{12}\) → \(\frac{√3}{2}\)
sinØ = \(\frac{√3}{2}\) or 60°
The bearing of Z from X = [270 - 60]° → 210°
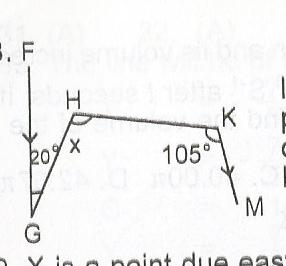
If in the diagram, FG is parallel to KM, find the value of x
75o
95o
105o
125o
Correct answer is B
No explanation has been provided for this answer.
\(\frac{800\pi}{9}\)km
\(\frac{800\sqrt{3\pi}}{9}\)km
800\(\pi\) km
800\(\sqrt{3\pi}\) km
Correct answer is C
Angular difference (\(\theta\))= 25° + 20° = 45°
\(\alpha\) = common latitude = 60°
\(S = \frac{\theta}{360°} \times 2\pi R \cos \alpha\)
\(S = \frac{45°}{360°} \times 2 \pi \times 6400 \times \cos 60°\)
= \(\frac{6400\pi}{8} = 800\pi km\)
JAMB Subjects
Aptitude Tests