JAMB Mathematics Past Questions & Answers - Page 111

551.

The cumulative frequency curve represents the ages of ages of students in a school. What age group do 70% of the students belongs?

17.5 - 20.5

16.5 - 19.5

15.5 - 19.5

15.5 - 18.5

View answer & explanation

Correct answer is A

No explanation has been provided for this answer.

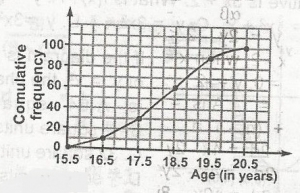
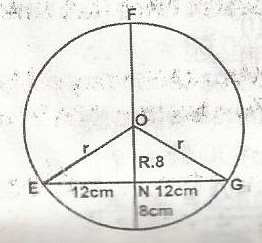
552.

If the diagram is the graph of y = x², the shaded area is

64 square units

\(\frac{126}{4}\) square units

32 square units

\(\frac{64}{3}\) square units

View answer & explanation

Correct answer is D

A = \(\int^{x = 4}_{x = 0}\) ydx = \(\int^{4}_{0}\) x²dx = [\(\frac{x^3}{3} + c]^{4}_{0}\)

= \(\frac{64}{3}\) square units

553.

In the diagram, EFGH is a circle centre O. FH is a diameter and GE is a chord which meets FH at right angle at the point N. If NH = 8cm and EG = 24cm, calculate FH

32cm

26cm

20cm

16cm

View answer & explanation

Correct answer is B

\(\bigtriangleup\)OEN = \(\bigtriangleup\)OGN

OE = OG = r(radii)

(r - 8)² + 12² = r²

r² - 16r + 64 + 144 = r²

16r = 64 + 144 = r²

16r = 64 + 144 = 208

\(\frac{208}{16}\) = 13cm

FH = 2FO = 2OH = 2R

= 2 x 13cm

= 26cm

554.

In the diagram, If < RPS = 50^o, < RPQ = 30^o and pq = QR, Find the value of < prs

50^o

60^o

70^o

80^o

View answer & explanation

Correct answer is C

< QPR = < PRQ = 30^o (PQ = QR)

< SPQ + < QRS = 180^o (supplementary)

80 + < QRS = 180^o = < QRS = 180^o - 80^o = 100^o

< QRP + < PRS = 100^o = 30^o + < PRS = 100^o

= < PRS = 100^o - 30^o = 70^o

555.

The grades of 36 students in a class test are as shown in the pie chart. How many student has excellent?

View answer & explanation

Correct answer is A

degree of excellent in the pie-chart = 360^o - (90^o + 120^o + 80^o) = 360^o - 290^o = 70^o

= 360^o = 36 students

70 = \(\frac{70^o}{360^o}\) x 36 = 7