Processing math: 18%

JAMB Mathematics Past Questions & Answers - Page 104

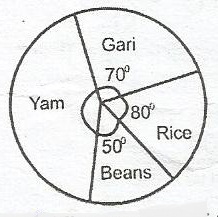
516.

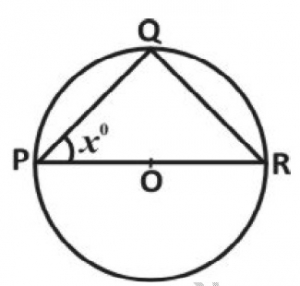
In the diagram above, PQR is a circle centre O. If < QPR is x°, find < QRP.

x°

(90 – x)°

(90 + x)°

(180 – x)°

View answer & explanation

Correct answer is B

< PQR = 90° (angle in a semi-circle) < QRP = (90 - x)°

517.

In diagram above, QR//TU, < PQR = 80° and < PSU = 95°. Calculate < SUT.

15^o

25^o

30^o

80^o

View answer & explanation

Correct answer is A

< PQR = < PTU = 80°

< TSU = 85°

x = 180° - (80° + 85°)

= 15°

518.

The shaded region above is represented by
the equation

y ≤ 4x + 2

y ≥ 4x + 2

y ≤ -4x + 4

y ≤ 4x + 4

View answer & explanation

Correct answer is C

Equation of the line

$\frac{y - 4}{x - 0} = \frac{0 - 4}{1 - 0}$

$\frac{y - 4}{x} = \frac{-4}{1}$

$\therefore -4x = y - 4$

$y = -4x + 4$

$\therefore \text{The shaded portion = } y \leq -4x + 4$

519.

The pie chart above shows the monthly distribution of a man's salary on food items. If he spent N8,000 on rice, how much did he spent on yam?

N42,000

N18,000

N16,000

N12,000

View answer & explanation

Correct answer is C

Angle of sector subtended by yam

= 360^o - (70 + 80 + 50)^o

= 360^o - 200^o

= 160^o

But $\frac{80^o}{360^o}$ x T = 8000

T = $\frac{8000 \times 360^o}{80^o}$

= N36,000

Hence the amount spent on yam = $\frac{160^o}{260} \times N36,000$

= N16,000

520.

in the figure above, what is the equation of the line that passes the y-axis at (0,5) and passes the x-axis at (5,0)?

y = x + 5

y = -x + 5

y = x - 5

y = -x - 5

View answer & explanation

Correct answer is B

(x1, y1) = (0,5)

(x2, y2) = (5, 0)

Using $\frac{y - y_1}{y_2 - y_1} = \frac{x - x_1}{x_2 - x_1}$

$\frac{y - 5}{0 - 5} = \frac{x - 0}{5 - 0}$

$\frac{y - 5}{-5} = \frac{x}{5}$

5(y - 5) = -5x

y - 5 = -x

x + y = 5

y = -x + 5