Loading [MathJax]/jax/output/HTML-CSS/jax.js

Home / Aptitude Tests / Mathematics / Find the area of the...

Find the area of the shades segments in the figure
...

Find the area of the shades segments in the figure

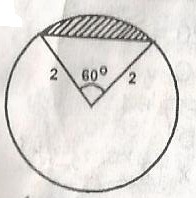
Find the area of the shades segments in the figure

A.

$\sqrt{3}$

B.

4 $\pi - \sqrt{3}$

C.

- $\frac{2}{3} \pi$

D.

$\frac{2\pi}{3}$ -3

View answer & explanation

Correct answer is D

Area of section = $\frac{60^o}{360^o}$ x 11r²

= $\frac{60}{360} \times \pi \times 2^2$

= $\frac{1}{6}$ x 4

= $\frac{4\pi}{6}$

= $\frac{2\pi}{3}$

Area of triangle = $\frac{1}{2x}$

= 2 x 28.......60

Segment Area = Area of section - Area of triangle

= $\frac{2\pi}{3}$ -3

See all Mathematics questions & answers

See more JAMB questions & answers

Similar Questions

Solve the equation x2 - 3x - 10 = 0...

In the figure, PQ and QR are chords of the circle PQR. QRS is a straight line and PR is equal to RS,...

If log520 = x, find x...

In the figure, $\bigtriangleup$ PQT is isosceles. PQ = QT, SRQ = 35o, TPQ = 20o and PQR is a straig...

Simplify $\sqrt{48}$ - $\frac{9}{\sqrt{3}}$ + $\sqrt{75}$ ...

In the figure, XYZ is a triangle with XY = 5cm, XZ = 2cm and XZ is produced to E making the angle < ...

Solve the equation 3x2 + 6x - 2 = 0 ...

The goals scored by 40 football teams from three league divisions are recorded below No...

Find the value of x in the equation 3x $^2$ - 8x - 3 = 0...

Solve: 6(x - 4) + 3(x + 7) = 3 ...

Aptitude Tests

Spatial Reasoning Questions and Answers

Nigeria General Knowledge Questions and Answers

Abstract Reasoning Questions and Answers

Microsoft Office Questions and Answers

Puzzles Questions and Answers

Personality Test

Verbal Reasoning Questions and Answers

Logical Reasoning Questions and Answers

Typing Speed and Accuracy Test

Numerical Reasoning Questions and Answers

Data Interpretation Questions and Answers

Quantitative Reasoning Questions and Answers

Situational Judgement Questions and Answers

View latest jobs in Nigeria today