How good are you with figures and formulas? Find out with these Mathematics past questions and answers. This Test is useful for both job aptitude test candidates and students preparing for JAMB, WAEC, NECO or Post UTME.
3\(\sqrt{3}\)
\(\frac{3(\sqrt{3} - 1)}{\sqrt{3} + 1}\)
\(\frac{(\sqrt{3} - 1)}{\sqrt{3} + 1}\)
\(\frac{3 - 1}{\sqrt{3} + 1}\)
Correct answer is B
\(\frac{x + 3}{sin 60^o}\) = \(\frac{x}{sin 306o}\)
3sin 30o = x sin 60o - x sin 30o
= x(sin 60o - sin 30o)
but sin 30o = \(\frac{1}{2}\)
sin 60o = \(\frac{3}{2}\) = \(\frac{3(\sqrt{3} - 1)}{\sqrt{3} + 1}\)
PQ is parallel to RS. Calculate the value of x.
20o
40o
60o
80o
100o
Correct answer is B
< D = 180o - 100v
= 80o (< on a str. line)
< s = 60o - alternate angle
x = 180o - (80o + 60o)
180o - 140o = 40o
In the parallelogram PQRS, PE is perpendicular to QR. Find the area of the parallelogram.
60cm2
65cm2
72cm2
132cm2
156cm2
Correct answer is D
By Pythagoras, PE\(^2\) = 12\(^2\) - 5\(^2\)
= 144 - 25 = 119
h = PE\(^2\) = √119 = 10.9 ≈ 11cm,
Area of 11gm = b x h
QR = b =(5 + 7)cm = 12cm
area = 12 x 11
= 132cm\(^2\)
\(\frac{5}{9}\)k2 sq. units
\(\frac{1}{3}\)k2 sq. units
\(\frac{8}{9}\)k2 sq. units
\(\frac{7}{9}\)k2 sq. units
\(\frac{2}{3}\)k2 sq. u
Correct answer is A
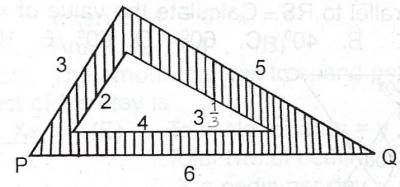
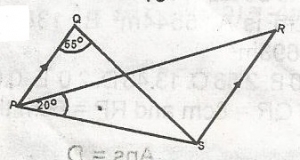
Area of shaded portion = Area of triangle PQR - Area of inner triangle
Area of triangle given 3 sides a, b, c = \(\sqrt{s(s - a)(s - b)(s - c)}\)
where \(s = \frac{a + b + c}{2} \)
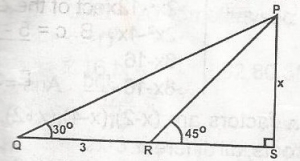
Area of PQR :
\(s = \frac{3 + 5 + 6}{2} = \frac{14}{2} = 7\)
Area = \(\sqrt{7(7 - 3)(7 - 5)(7 - 6)}\)
= \(\sqrt{7(4)(2)(1)} = \sqrt{56}\)
\(\implies K^{2} = \sqrt{56}\)
Area of inner triangle :
\(s = \frac{2 + 4 + \frac{10}{3}}{2} = \frac{14}{3}\)
Area = \(\sqrt{\frac{14}{3} (\frac{14}{3} - 2)(\frac{14}{3} - 4)(\frac{14}{3} - \frac{10}{3})}\)
= \(\sqrt{\frac{14}{3} (\frac{8}{3})(\frac{2}{3})(\frac{4}{3})}\)
= \(\sqrt{\frac{896}{81}}\)
= \(\sqrt{\frac{16}{81}} \times \sqrt{56}\)
= \(\frac{4}{9} K^{2}\)
\(\therefore \text{The area of the shaded portion} = K^{2} - \frac{4}{9}K^{2} = \frac{5}{9}K^{2}\)
45o
35o
40o
30o
42o
Correct answer is D
PSR = 65o x 2
= 130o
PRS = 180o - (130o + 20o)
= 30o