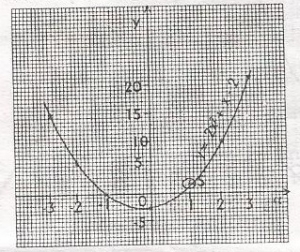
The graph given is for the relation y = 2x2 + x - 1. Find the minimum value of y
0.00
-0.65
-1.25
-2.10
Correct answer is C
No explanation has been provided for this answer.
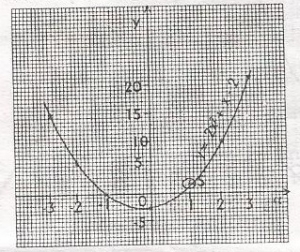
The graph given is for the relation y = 2x2 + x - 1.What are the coordinates of the point S?
(1, 0.2)
(1, 0.4)
(1, 2.0)
(1, 40)
Correct answer is C
No explanation has been provided for this answer.
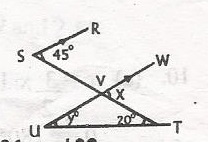
20
25
45
65
Correct answer is B
xo + zo = 180o (sum of < on str. line)
45o + zo = 180o
zo = 180o - 45o
z = 135o
z + yo + 20o = 180o (sum of < s in \(\bigtriangleup\))
135o + yo + 20o = 180o
yo + 155o = 180o
yo + 180o - 155o
yo = 25o
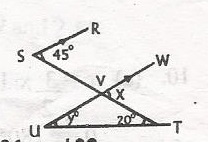
20
45
65
135
Correct answer is B
From the diagram SR//UW given that < RSV = 45o and < WVT = xo
< WVT = 45o(corresponding angle)
xo = 45

In the diagram, PQR is a straight line, (m + n) = 120o and (n + r) = 100o. Find (m + r)
110o
120o
140o
160o
Correct answer is C
No explanation has been provided for this answer.
WAEC Subjects
Aptitude Tests