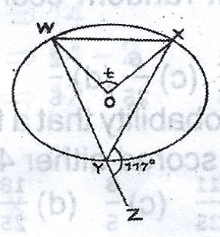
Find the value of t in the diagram
63o
117o
126o
234o
Correct answer is C
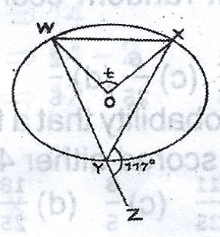
In the diagram,
α = 180o - 177o (angles on a straight line)
α = 63o
t = 2 x 63o (angle at centre = 2 x angle at circum)
= 126o
Find the mean deviation of 20, 30, 25, 40, 35, 50, 45, 40, 20 and 45
8
9
10
12
Correct answer is B

Mean = ∑fx∑f
= 35010
= 35
= ∑f|d|∑f
= 9010
= 9
Find the median of 2, 1, 0, 3, 1, 1, 4, 0, 1 and 2
0.0
0.5
1.0
1.5
Correct answer is C
First arrange the numbers in ascending order of magnitude; 0, 0, 1, 1, 1, 1, 2, 2, 3, 4
Counting from the right, the fifth number is 1
Counting from the left, the fifth number is 1
Hence, median = frac1+12
= 22
= 1
If P and Q are two statements, under what condition would p|q be false?
If p is true and q is true
If p is true and q is false
If p is false and q is false
If p is false and q is true
Correct answer is B
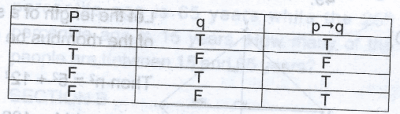
From the truth table above, p → q would be false if p is true and q is false
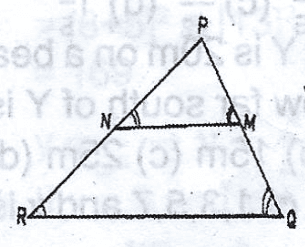
In the diagram, which of the following ratios is equal to |PN||PQ|?
|PN||PR|
|PM||PQ|
|PM||PR|
|PR||PQ|
Correct answer is C
|PN||PQ| = |PM||PR|
WAEC Subjects
Aptitude Tests