1,592.
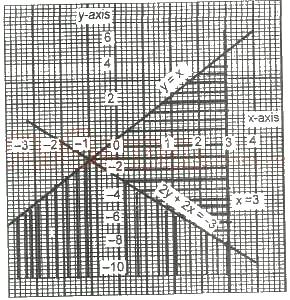

The area shaded with horizontal lines is the solution set of the inequalities;
A.
y ≥ x, y + 3 ≥ 2x, x ≤ 3
B.
y ≤ x, y + 2x ≥ -3, x ≤ 3
C.
y ≤ -x, y + 2x ≤ 3, x ≥ -3
D.
y ≥ -x, y + 3 ≤ 2x, x ≥ -3
E.
y ≤ x, y ≤ 2x - 3, x ≥ 3
Correct answer is B
No explanation has been provided for this answer.
1,594.
Let J be the set of positive integers, If H = {x: x∈J, x^2 < 3 and x ≠ 0}, then
A.
H = {1}
B.
H is an infinite set
C.
H = {0, 1, 2}
D.
H = {}
E.
J ≤ H
Correct answer is A
H = {x: x is a positive integer, x^2 < 3 and x \neq 0}
H = {1}
1,595.
Find the sum of the first five terms of the G.P 2,6, 18 ....
A.
484
B.
243
C.
242
D.
130
E.
121
Correct answer is C
S_{n} = \frac{a(r^n - 1)}{r - 1}
a = 2; r = 6/2 = 3.
S_{5} = \frac{2(3^5 - 1)}{3 - 1}
= 243 - 1 = 242
WAEC Subjects
Aptitude Tests
