Processing math: 100%

WAEC Mathematics Past Questions & Answers - Page 29

141.

Which of the following is not a sufficient condition for two triangles to be congruent?

AAS

SSS

SAS

SSA

View answer & explanation

Correct answer is D

No explanation has been provided for this answer.

142.

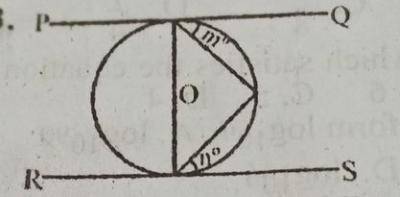
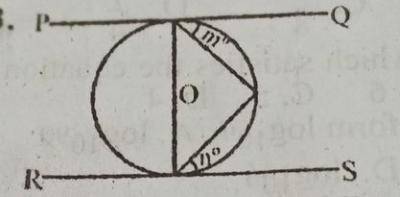
In the diagram, O is the center of the circle. $\overline{PQ}$ and $\overline{RS}$ are tangents to the circle. Find the value of (M + N).

120 $^o$

90 $^o$

75 $^o$

60 $^o$

View answer & explanation

Correct answer is B

m + n + 90 $^o$ + 180 $^o$ (sum of angle in a $\triangle$ )

m + n = 180 $^o$ - 90 $^o$

m + n =90 $^o$

143.

x	6.20	6.85	7.50
y	3.90	5.20	6.50

The points on a linear graph are as shown in the table. Find the gradient (slope) of the line.

2 $\frac{1}{2}$

$\frac{1}{2}$

View answer & explanation

Correct answer is B

Gradient = $\frac{y_2 - y_ 1}{x_2 - x_1}$

= $\frac{5.20 - 3.90}{6.85 - 6.20}$

= $\frac{1.3}{0.5}$

= 2

144.

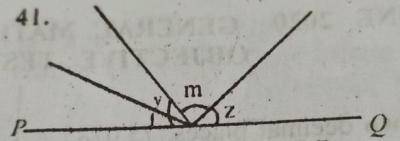
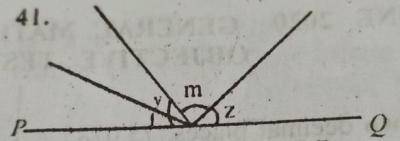
In the diagram, PQ is a straight line. If m = $\frac{1}{2}$ (x + y + z), find value of m.

45 $^o$

60 $^o$

90 $^o$

100 $^o$

View answer & explanation

Correct answer is B

x + y + m + 2 = 180 $^o$

$\frac{x + y + 2}{2} + \frac{m}{2}$ = 90 $^o$

m + $\frac{m}{2} = 90^o$

3m = 2 x 90 $^o$

$\frac{3m}{3} = \frac{180^o}{3}$

m = 60 $^o$

145.

A box contains 12 identical balls of which 5 are red, 4 blue, and the rest are green. If two balls are selected at random one after the other with replacement, what is the probability that both are red?

$\frac{25}{144}$

$\frac{5}{33}$

$\frac{5}{6}$

$\frac{103}{132}$

View answer & explanation

Correct answer is A

Pr(RR) = $\frac{5}{12}$ = $\frac{5}{12} \times \frac{5}{12} = \frac{25}{144}$

WAEC Subjects

Literature in English

Islamic Religious Knowledge

Christian Religious Knowledge

Aptitude Tests

Verbal Reasoning Questions and Answers

Typing Speed and Accuracy Test

Data Interpretation Questions and Answers

Puzzles Questions and Answers

Quantitative Reasoning Questions and Answers

Abstract Reasoning Questions and Answers

Situational Judgement Questions and Answers

Personality Test

Numerical Reasoning Questions and Answers

Spatial Reasoning Questions and Answers

Nigeria General Knowledge Questions and Answers

Logical Reasoning Questions and Answers

Microsoft Office Questions and Answers

See all →

View latest jobs in Nigeria today