\(9\pi cm^2\)
\(15\pi cm^2\)
\(16\pi cm^2\)
\(20\pi cm^2\)
Correct answer is B
Curved surface area or a cone \(=\pi rl\)
from the information \(l^2 = 4^2 + 3^2 = 16+9\\
l = \sqrt{25} = 5; ∴ C.S.A\hspace{1mm} = \frac{22}{7}\times 3 \times 5\\
Since \frac{22}{7}=\pi ∴ C.S.A\hspace{1mm} =\hspace{1mm}15\pi\)
\(5\frac{1}{3}cm^3\)
\(10\frac{2}{3}cm^3\)
\(16cm^3\)
\(32cm^3\)
Correct answer is B
No explanation has been provided for this answer.

8m
9m
10m
13m
Correct answer is A
Given, Length of minor arc = 5.5m
Angle subtended by minor arc = 360° - 210° = 150°
\(\therefore 5.5 = \frac{150}{360} \times 2 \times \frac{22}{7} \times r \)
\(\frac{55r}{21} = 5.5\)
\(r = \frac{5.5 \times 21}{55}\)
r = 2.1m
Length of major arc = \(\frac{210}{360} \times 2 \times \frac{22}{7} \times 2.1\)
= \(7.7m \approxeq 8m\) (to the nearest metre)

In the diagram, PQ is the diameter of the circle and ∠PRS = 58°. Find ∠STQ.
29o
32o
42o
53o
Correct answer is B
In \(\Delta\) PRQ, < SRQ = 90° - 58°
< SRQ = < QTS = x (angles in the segment)
\(\therefore\) x = 32°
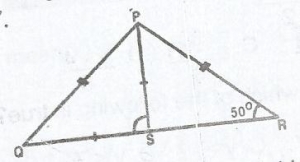
51o
64o
77o
103o
Correct answer is D
cos\(\alpha\) = \(\frac{10}{16}\) = 0.625
\(\alpha\) = cos-1(0.625)
= 51.3o
∠PQR = 2\(\alpha\)
= 2(51.3o)
= 102.6
= 103o
WAEC Subjects
Aptitude Tests