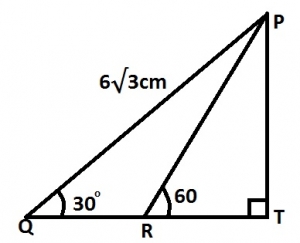
0.3cm
\(\frac{\sqrt{3}}{2}cm\)
3cm
\(3\sqrt{3}cm\)
Correct answer is C
In \(\Delta\) QPT,
\(\frac{PT}{6\sqrt{3}} = \sin 30°\)
PT = \(6\sqrt{3} \times \frac{1}{2} = 3\sqrt{3} cm\)
In \(\Delta\) RPT,
\(\frac{PT}{RT} = \tan 60°\)
\(\frac{3\sqrt{3}}{RT} = \tan 60°\)
\(RT = \frac{3\sqrt{3}}{\sqrt{3}} = 3 cm\)
If q oranges are sold for t Naira, how many oranges can be bought for p naira?
\(\frac{p}{2}t\)
\(\frac{qt}{p}\)
\(\frac{q}{pt}\)
\(\frac{pq}{t}\)
Correct answer is D
q oranges = t naira
1 naira = \(\frac{q}{t}\)
p naira = \(p(\frac{q}{t})\)
= \(\frac{pq}{t}\) oranges
(2a-b)(2n-m)
(2a+b)(m-2n)
(2a-b)(m+2n)
(2a-b)(m-2n)
Correct answer is C
m(2a - b) - 2n(b - 2a)
= m(2a - b) - (-2n)(2a - b)
= m(2a - b) + 2n(2a - b)
= (m + 2n)(2a - b)
Simplify \(3\sqrt{12} + 10\sqrt{3} - \frac{6}{\sqrt{3}}\)
\(7\sqrt{3}\)
\(10\sqrt{3}\)
\(14\sqrt{3}\)
\(18\sqrt{3}\)
Correct answer is C
\(3\sqrt{12} + 10\sqrt{3} - \frac{6}{\sqrt{3}}\)
= \(3(\sqrt{4 \times 3}) + 10\sqrt{3} - (\frac{6}{\sqrt{3}})(\frac{\sqrt{3}}{\sqrt{3}})\)
= \(6\sqrt{3} + 10\sqrt{3} - 2\sqrt{3}\)
= \(14\sqrt{3}\)
The volume of a cylinder of radius 14cm is 210cm3. What is the curved surface area of the cylinder?
15cm2
30cm2
616cm2
1262cm2
Correct answer is B
\(V = \pi r^2 h\\
210 = \frac{22}{7} \times 14^2 \times h \\
h = \frac{210}{22 \times 28}\)
Curved surface area \(= 2r\pi h\\
= 2 \times \frac{22}{7} \times 14 \times \frac{210}{22 \times 26} = 30cm^2\)
WAEC Subjects
Aptitude Tests