Simplify 712−(212+3)÷1612and correct your answer to the nearest whole number
33
8
7
0
Correct answer is C
712−(212+3)÷1612; 152−(52+31)÷332
152−(5+62)÷332; 152−112÷332
112 ÷ 332 = 112 * 233
= 1133 or 13 (when simplified)
152 - 13 = 436
7.166
The nearest whole number is 7
m12
12m
12m+12
12m−12
Correct answer is C
Prob. (a girl) =NumberofgirlsNumberofboysandgirlsinclass=12m+12
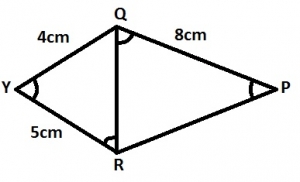
In the diagram, RˆPQ=QˆRY,PˆQR=RˆYQ,|QP|=3cm|QY|=4cmand|RY|=5cm.Find|QR|
2.0cm
2.5cm
6.4cm
10.0cm
Correct answer is C
PRQR=QRQY=QPYR (SSS Congruence)
QR4=85
QR=4×85
= 6.4 cm
A school girl spends 14 of her pocket money on books and 13 on dress. What fraction remains?
56
712
512
16
Correct answer is C
Let the girls pocket money be rep. by x. The amount spent on books = 14ofx=x4
The amount spent on dress =13ofx=x3
∴The fraction that remains = x1−(x4+x3)3x+4x12;=12x−7x12=5x12=512
Solve for t in the equation 34t+13(21−t) = 11,
913
95
5
935
Correct answer is D
\frac{3}{4}t+\frac{1}{3}(21-t) = 11; \frac{3t}{4} + \frac{7}{1} - \frac{t}{3} = \frac{11}{1}\\ \frac{3 \times 3t + 7\times 12 – 4 \times t = 11 \times 12}{12}\\ 9t + 84 – 4t = 132; 5t = 132 – 84\\ 5t = 48; t = \frac{48}{5} = 9\frac{3}{5}
WAEC Subjects
Aptitude Tests