Given that sin (5x-28)° = cos (3x-50)", 0°≤ x ≤ 90°, find the value of x.
39
32
21
14
Correct answer is B
using the trial method by inserting each option in the equation.
Inserting 21º: sin([5 x 21] - 28) = cos([3 x 21] - 50)
sin(105 - 28) = cos (63 - 50)
sin 77º = cos 13º
where:
sin 77º = 0.9744
cos 13º = 0.9744
70º
47º
19º
8º
Correct answer is A
The angle of elevation
= Tan θ = \(\frac{opp}{adj}\)
Tan θ = \(\frac{12+14}{10}\)
Tan θ = \(\frac{26}{10}\)
θ = Tan\(^{-1}\)(2.6)
θ ≈ 70º
The length of a rectangle is 10 cm. If its perimeter is 28 cm, find the area
30cm\(^2\)
40cm\(^2\)
60cm\(^2\)
80cm\(^2\)
Correct answer is B
perimeter = 2( length + breadth)
→ 28 = 2 (10+ b)
14 - 10 = b
b = 4
Area = length x breadth
10 x 4 → 40cm\(^2\)

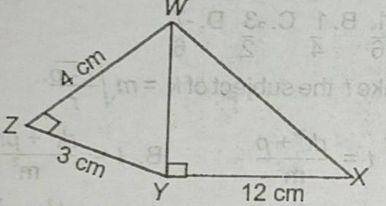
In the diagram, ∠ZWZY and WYX are right angles. Find the perimeter of WXYZ.
30cm
32cm
35cm
37cm
Correct answer is B
In ΔWYZ:
hyp\(^2\) = adj\(^2\) + opp\(^2\)
hyp\(^2\) =3\(^2\) + 4\(^2\) → 9 + 16
hyp\(^2\) = 25
hyp = 5
In ΔWXY:
hyp\(^2\) = adj\(^2\) + opp\(^2\)
hyp\(^2\) = 12\(^2\) + 5\(^2\) = 144 +25
hyp\(^2\) = 169
hyp = 13
the perimeter of WXYZ. = 3+4+12+13 → 32cm
126.5cm\(^2\)
165.0cm\(^2\)
212.0cm\(^2\)
214.5cm\(^2\)
Correct answer is D
The surface area of an open-top cylinder = πr(r + 2h),
where 'r' is the radius and 'h' is the height of the cylinder.
= \(\frac{22}{7}\) * 3.5 (3.5 + 2 * 8)
= 11 (3.5 + 16) → 11 (19.5)
= 214.5cm\(^2\)
WAEC Subjects
Aptitude Tests