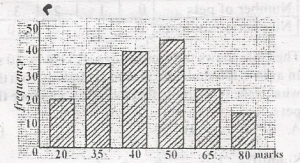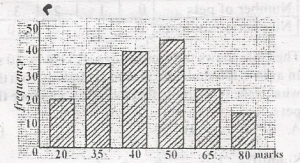
5513%
60%
65%
6623%
Correct answer is D
Percentage of students with marks ranging from 35 to 50 = f35+f40+f50∑f
= 35+40+4520+35+40+45+25+15 x 100%
= 120180 x 100%
= 6623%

In the diagram, < PSR = 220o, < SPQ = 58o and < PQR = 41o. Calculate the obtuse angle QRS.
90o
100o
121o
60o
Correct answer is C
Joining SQ. In △ SPQ,
(22o + a) + 55o + (41o + b) = 180o
121o + a + b = 180o
a + b = 180 - 121
a + b = 59o.....(1)
In △ SRPQ; R + a + b = 180o
R + 59o = 180o
(in (1), a + b = 59o)
R = 180 - 59
R = 121o
332
73
53
516
Correct answer is D
Pr. (winning 100m race) = 18
Pr. (losing 100m race) = 1 - 18 = 78
Pr. (winning high jump) = 14
Pr. (losing high jump ) = 1 - 14 = 34
Pr. (winning only one) = Pr. (Winning 100m race and losing high jump) or Pr.(Losing 100m race and winning high jump)
= (18×34) + (78×14)
= 332+732
= 1032
= 516
The mean of the numbers 2, 5, 2x and 7 is less than or equal to 5. Find the range of the values of x
x ≤ 3
x ≥ 3
x < 3
x > 3
Correct answer is A
mean ≤ 5; 2+5+2x+74 ≤ 5
= 14+2x4≤5
= 14 + 2x ≤ 5 x 4
14 + 2x ≤ 20 ; 2x ≤ 20 - 14
2x ≤ 20 - 14
2x ≤ 6
x ≤ 62
x ≤ 3
WAEC Subjects
Aptitude Tests