Calculate the area of an equilateral triangle of side 8cm
8√3
16
4√3
16√3
Correct answer is D
An equilateral triangle has all sides equal and all angles equal as 600
Area = 12 absinθ
Area = 12 x 8 x 8 x sin60
= 12 x 64 x √32
= 16√3 cm2
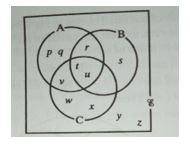
Which one of the following gives the members of the set A1 n B n C?
Φ
{s}
{t, u}
{y, z}
Correct answer is A
A1 = Elements in the universal set but not in A = {s, w, x, y, z} B = {r, s. t, u} C = {t, u, v, w, x} A1 n B n C = elements common to the three sets = none = empty set = Φ
if y = 23five + 101three find y leaving your answer in base two
1110
10111
11101
111100
Correct answer is B

First we convert the numbers to base ten
23five= 2 x 51 + 3 x 50
= 10 + 3 = 13
101five = (1 x 32) + (0 x 31) + (1 x 30)
= 9 + 0 + 1 = 10
So, y = 13 + 10 = 23
To convert 23 to base 2 (as in the diagram above)
Y = 23
= 10111five
Answer is B
From a point P, Q is 5km due West and R is12km due South of Q. Find the distance between P and R.
5km
12km
13km
17km
Correct answer is C
Using Pythagoras theorem
PR2 = 52 + 122
25 + 144 = 169
PR = √(169)= 13km
JAMB Subjects
Aptitude Tests