If sin θ = - 35 and θ lies in the third quadrant, find cos θ
45
- 54
54
- 45
Correct answer is D
Where sin θ = opphyp → −35
opp = -3, hyp = 5
using pythagoras formula
hyp2 = adj2 + opp2
adj2 = hyp2 - opp2
adj2 = 52 - 32 → 25 - 9
adj2 = 16
adj = 4
cos θ = adjhyp → 45
In third quadrant: cos θ is negative → - 45
If the mean of 2, 5, (x+1), (x+2), 7 and 9 is 6. Find the median
5.5
5
6.5
6
Correct answer is C
Firstly; solving for x
6 = 2+5+x+1+x+2+7+96
cross multiply to have:
6 * 6 = 2 + 5 + x+1 + x+2 + 7 + 9
36 = 2x + 26
36 - 26 = 2x
10 = 2x
x = 5
Median = 7+62
→ 6.5
If a fair coin is tossed twice, what is the probability of obtaining at least one head?
0.25
0.75
0.5
0.33
Correct answer is B
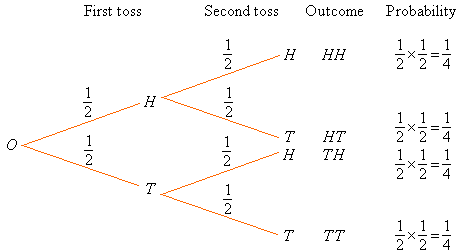
No explanation has been provided for this answer.
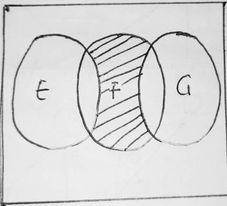
The shaded portion in the venn diagram above represents?
F - (E n F) - (G n F)
E' n F n G'
E u F u G
F
Correct answer is B
-The shaded/stripped portion in the diagram is set F only.
-This implies that sets E and G though present in the Universal set, are not included in the expression.
-Mathematically; we have E' n F n G'
Solve for k in the equation 18k+2 = 1
2
-4
-2
4
Correct answer is C
(8−1)k+2 = (80)
base 8 cancel out on both sides
-1(k+2) = 0
-k -2 = 0
: k = -2
JAMB Subjects
Aptitude Tests