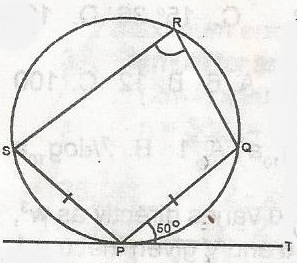
In the diagram, if PS = SR and PQ||SQ, what is the size of PQR?
25o
50o
55o
65o
75o
Correct answer is B
Explanation
< PQR + , PSR 180(Opposite angles in a Quadrilateral are supplementary)< PQR + 130o = 180
< PQR = 180 - 130
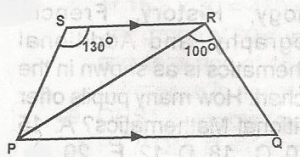
In the figure, PQR is a straight line. Find the values of x and y
x = 22.5o, y = 33.75o
x = 15o, y = 52.5o
x = 22.5o, y = 45.0o
x = 56.25o, y = 11.5o
Correct answer is A
\(\frac{3}{2}\)x + 3y + 45° = 180°
3x + 6y + 90° = 360°
3x + 6y = 270.......(i) x 2,
5x + y + y = 180°
5x + 2y = 180° ... (ii) x 6
6x + 12y = 540 ... (iii)
30x + 12y = 1080 ... (iv)
eqn(iv) - eqn(iii)
24x = 540
x = 22.5° and y = 33.75°
11o
19\(\frac{1}{3}\)o
33\(\frac{1}{3}\)o
91\(\frac{1}{3}\)o
91o
Correct answer is B
Adding the values of all the items together, it gives 70
Okro sector = \(\frac{145}{270}\) x \(\frac{360^o}{1}\)
= 19.33o
= 19\(\frac{1}{3}\)o
50o
40o
110o
80o
100o
Correct answer is E
< SPQ = 80o
< SPQ + < SRQ = 180(Supplementary)
80 +
< QRS = 180o - 80o
= 100o
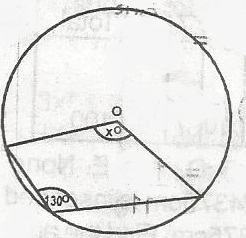
If O is the centre of the circle in the figure, find the value of x
50
260
100
65
130
Correct answer is C
From the diagram; The value of x = 360o - 2(130o)
= 360 - 260
= 100o
JAMB Subjects
Aptitude Tests