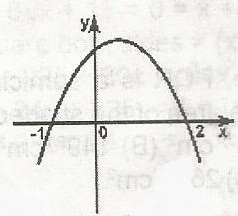
What is the equation of the quadratic function represented by the graph?
y = x2 + x - 2
y = -x2 - x + 2
y = x2 - x - 2
y = -x2 - x + 2
Correct answer is C
The required equation is y = x2 - x - 2
i.e. B where the graph touches the graph touches the x-axis y = 0
x2 - x - 2 = 0 = (x + 1)(x - 2) = 0
Hence roots of the equation are -1 and 2 as shown in the graph

In the figure, PQ is a parallel to ST and QRS = 40o. Find the value of x
55o
60o
65o
75o
Correct answer is A
From the figure, 3x + x - 40o = 180o
4x = 180o + 40o
4x = 220o
x = \(\frac{220}{4}\)
= 55o
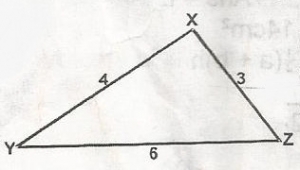
In \(\bigtriangleup\)XYZ, determine the cosine of angle Z.
\(\frac{3}{39}\)
\(\frac{29}{36}\)
\(\frac{14}{5}\)
\(\frac{7}{5}\)
Correct answer is B
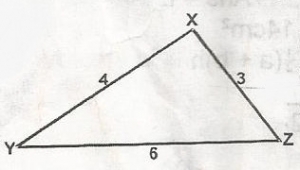
cos z = \(\frac{y^2 + x^2 -z^2}{2yx}\)
= \(\frac{9 + 36 - 16}{2(3)(6)}\)
= \(\frac{29}{36}\)
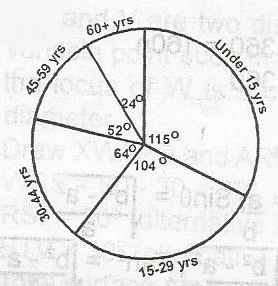
29 x 104
26 x 104
16 x 104
13 x 104
Correct answer is B
15 - 29 years is represented by 104o
Number of people in the group is \(\frac{104}{360}\) x 0.9m
= 260000 = 26 x 104
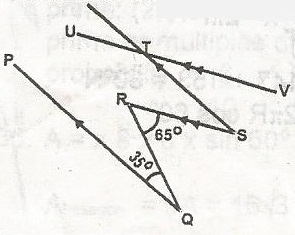
In the figure, PQ||ST, RS||UV. If PQR = 35o and QRS = 65o, find STV
30o
35o
55o
65o
Correct answer is A
Draw XW//PQ and ARW = 35o (alternative angle)
WRS = 60 - 30
= 30o
RSR = 30o (Alternative angle)
STV = 30o (Alternative angle)
JAMB Subjects
Aptitude Tests