Triangle SPT is the solution of the linear inequalities
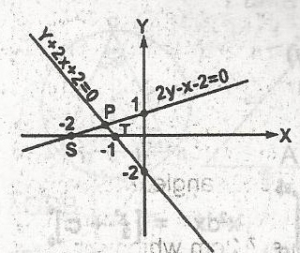
Triangle SPT is the solution of the linear inequalities
A.
2y - x - 2 ≤ 0, y + 2x + 2 ≥ 0, x ≥ 0
B.
2y - x - 2 ≥ 0, y + 2x + 2 ≤ 0, -2 ≥ x ≥ -1
C.
-2 ≥ x ≥ 2, y ≤ 0, y + 2x + 2 ≥ 0, x ≥ 0
D.
2y - x - 2 ≥ 0, y + 2x + 2 ≥ 0, y ≤ 0, x ≥ 0
Correct answer is C
No explanation has been provided for this answer.
Similar Questions
Simplify and express in standard form 0.00275×0.006400.025×0.08...
lf sin θ= −12, find all the values of θ between 0° and 360°....
In a triangle XYZ, < YXZ = 44° and < XYZ = 112°. Calculate the acute angle b...
Which of the following is not necessarily sufficient for the construction of a triangle? ...