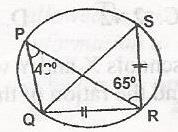
In the diagram, |SR| = |QR|. < SRP = 65^o and < RPQ = 48^o, find < PRQ

Similar Questions

If log$_{10}$ q = 2.7078, what is q? ...

Find the median of 5,9,1,10,3,8,9,2,4,5,5,5,7,3 and 6 ...

In the diagram, PR is a diameter, ∠PRQ = (3x-8)° and ∠RPQ = (2y-7)°. Find x in terms...

Find m such that (m + $\sqrt{3}$)(1 - $\sqrt{3}$)2 = 6 - 2$\sqrt{2}$...

Given that $\frac{1}{2} \log_{10} P = 1$, find the value of P....

The sum of two numbers is twice their difference. If the difference of the numbers is P, find the la...

The diagram above shows the shaded segment of a circle of radius 7cm. if the area of the triangle OX...

The lengths of the sides of a right angled triangle are (3x + 1)cm, (3x - 1)cm and xcm. Find x...

In a class of 80 students, every student had to study Economics or Geography or both Economics and G...

If N225.00 yields N27.00 in x years simple interest at the rate of 4% per annum, find x.  ...