In the diagram, O is the center of the circle QRS and&nbs...
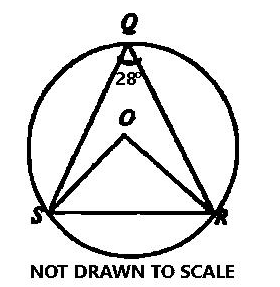
In the diagram, O is the center of the circle QRS and ∠SQR = 28°. Find ∠ORS.
560
280
760
620
Correct answer is D
∠SOR = 2 × 28° = 56° (angle at the centre is twice the angle at the circumference)
From ∆SOR
|OS| = |OR| (radii)
So, ∆SOR is isosceles.
∠ORS = 1800−5602=12402 ( base angles of isosceles triangle are equal)
∴ ∠ORS = 62°
Similar Questions
The probability of an event P is 34 while that of another event Q is 16. I...
The marks scored by 30 students in a Mathematics test are recorded in the table below: Scores...
Find the area, to the nearest cm2, of the triangle whose sides are in the ratio 2 : 3 : 4 and w...
In what number base is the addition 465 + 24 + 225 = 1050? ...
In the diagram, EFGH is a cyclic quadrilateral in which EH || FG, EG, and FH are chords. If < FHG...
Solve the equation 4/a + 1/5a = 3 ...
Solve the equations m2 + n2 = 29 m + n = 7 ...
Evaluate ∫(cos4x + sin3x)dx...
In the diagram, find PQ if the area of triangle PQR is 35cm2...