The chord ST of a circle is equal to the radius r of the ...
The chord ST of a circle is equal to the radius r of the circle. Find the length of arc ST
πr3
πr2
πr12
πr6
Correct answer is A
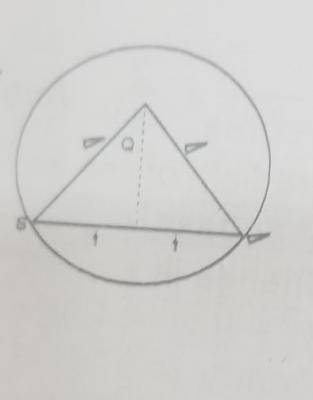
r2r Sin θ = 12
θ = sin−1 (12) = 30o = 60o
Length of arc (minor)
ST = θ360 x 2πr
60360×2π×r=π3
Similar Questions
How many three-digit numbers can be formed from 32564 without repeating any of the digits? ...
If \(N = \begin{pmatrix} 3 & 5 & -4 \\ 6 & -3 & -5 \\ -2 & 2 & 1 \end{pmatri...
In how many ways can 6 coloured chalks be arranged if 2 are the same colour? ...
If F = 95C + 32, find C when F = 98.6...
In the diagram above, M, N, R are points on the circle centre O. ∠ORN = 48° and &a...
Find the 4th term of an A.P, whose first term is 2 and the common difference is 0.5...
If sinθ cosθ, for 0o ≤ θ ≤ 360o, find the value of θ...
On a map, 1cm represent 5km. Find the area on the map that represents 100km2....
In the figure, PQ||ST, RS||UV. If PQR = 35o and QRS = 65o, find STV...