The velocity ratio of an inclined plane where angle of in...
The velocity ratio of an inclined plane where angle of inclination in θ is
A.
Sinθ
B.
Cosθ
C.
Tanθ
D.
1sinθ
Correct answer is D
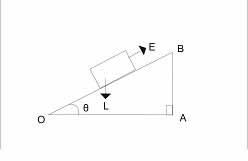
Consider an inclined plane shown below, as the effort moves along OB, the load moves is lifted up through a vertical height AB
V.R = distance moved by effortdistance moved by load = OBAB
But Sinθ = opphyp = ABOB
therefore; = OBAB = 1sinθ
Similar Questions
Which of the following statements about photoelectrons is correct? ...
A short response to time is obtained in liquid-in-glass thermometer when the ...
A cone is in unstable equilibrium, has its potential energy ...
When the human eye loses its power of accomodation, the defect is known as ...